Bonus Chasing, Earn Rates, and Variance

As promised in the last blog post, today's post will be about bonus clearing, its effect on your earn rate, and what that means for variance.

First, a very basic introduction to bonus clearing: many sites have bonuses that clear at a specific "multiplier" to the amount of money you have to clear. For example, River Belle (a Prima skin) currently has the following bonus, which will be used as the ongoing example throughout this post.

"50% up to $100, plus $25 if deposited via an ADM, clearing at 7x raked hands"

Wow - that's a mouthful. What this means in English is that they will give you 50 cents on the dollar (50%) for any money you deposit, and they will do this until their match reaches $100. They will give you an additional $25 if you deposit via Neteller or Firepay. Thus, if you deposit $200 via Neteller, they will throw $125 in your bonus account. This bonus will clear at 7x, which means you will have to play $125 x 7 = 875 raked hands for your bonus to be released to you. That's pretty much it. Some sites do things in terms of "points" to clear bonuses, but these can almost always be converted back into multipliers with appropriate massaging. Also, some sites release your bonus to you in "stages" instead of giving to you as one lump sum at the end - this does not affect the calculations in this post, but is nice to know.

So, in order to clear the $125 bonus, we have calculated that 875 raked hands are necessary. Next step is to determine how the site determines a "raked hand". At River Belle, a raked hand is any hand where you are dealt cards and the pot is raked at least $0.25. Other sites (such as Party Poker) count any hand which is raked (even at $0.05) as a raked hand, which has major implications on the bonus clearing rate. More on this later.

Obviously, if you play at a lower level (say, $0.25/$0.50) the pots will not be raked at $0.25 or more as often as if you play $2/$4. This implies you will need many more dealt-hands at a lower level to get a fixed number of raked-hands than you will at a higher level, and this needs to be taken care of in any calculation.

So, to take our above example, let's assume we decide to play $0.25/$0.50 to clear our $125. So far, my experience shows that about 20% of hands at that level are raked at $0.25 or above. Thus, in order to get in our 875 raked hands, we will need to play about (875 / 20%) = 4375 hands. This allows us to calculate two numbers:

• Our BB/100 just for clearing the bonus: $125 is 250 BB ($125/$0.50), and we need to play 4375 hands to earn that, so our BB/100 just from bonus clearing is (250 BB / 43.75 ) = 5.7 BB/100
• Our hourly earn rate: Assume 60 hands per hour per table. 4375 hands should take 72.9 hours of single-table play, thus meaning you earned $125 / 72.9 = $1.71 / hour per table.

We can do this calculation for a variety of levels, and a variety of bonus structures, and the result is the below table (click for larger image):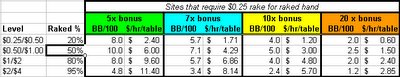

You can see our previous 5.7 BB/100 and $1.71/hr/table numbers in their appropriate spots in the table. A few things to notice about this table:

• In terms of BB/100 winrate, there seems to be a bit of a "sweet spot" around $0.50/$1.00 - it seems to be the "ideal" level to clear bonuses in terms of BB/100
• In terms of hourly rate, the larger limits are always better (to a point, as the next table will show)
• The winrate numbers are much larger at the lower levels than even a good 2 BB/100 player could generate via their play alone.

But the story gets better - check out the following table for sites (such as Party) that have a very low limit for what constitutes a raked hand (click for larger image):

Technical note: the usage of 95% in the above models for the higher levels assumes a "no flop / no drop" policy whereby if no flop is seen, no rake is taken. 95% corresponds to not seeing a flop once every two button revolutions, which seems about right for the low levels I've played so far. This number may actually have to be lowered as higher levels are reached, as the games may tighten up and blind steals may succeed more often than at the lower levels. Also note that as nearly every hand becomes raked, the hourly rate to clear your bonus becomes constant regardless of what level you play.

These numbers are HUGE for the lower levels, and are quite respectable even for the upper levels shown in the chart. Remember - this is just the clearing rate for the bonus. Any winrate you have from playing poker goes on top of this!

So, let's take our group of 10000 clones from the earlier post (2BB/100, SD=16BB/100) and put them on this RiverBelle bonus playing at $0.25/$0.50. Let's see how they stack up over the first 4375 hands against a group who isn't playing the bonus. The non-bonus players should win right around 88 BB (4375 * 2BB/100 / 100), so their bell curve should center around there. The bonus players should win the same but have an extra 250 BB ($125 / $0.50) for a total of 335 BB, so their curve should center around that. Cue the graph (again, click for larger image):

Perhaps as expected, the result of the bonus is to merely shift the bell curve to the right by 250 BB without increasing its width (a measure of standard deviation). This is as a result of the fact that adding your bonus does notincrease your Standard Deviation at all - in effect, your bonus is the equivalent of a 5.7 BB/100, 0 SD player playing "alongside" you. Ignore the fact that the curves look slightly different in shape - this is an artifact of the fact that the bonus is not an exact multiple of the bin size.

But look at what this "trivial" result means for the "unlucky" low-end of the tail! Whereas over 20% of the non-bonus players would have lost money over these 4375 hands, only 6 of the bonus players have lost money! (the bar representing these 6 players is actually too short to see on the diagram - the first bar you see is the 91 players who are up between 0 and 88 BB). The breakdown of that 20% group is:

• 1573 players lost between 0 and 88 BB
• 425 players lost between 88 and 176 BB
• 62 players lost more than 176 BB

So which group would you rather be in? I know I'd rather take a 0.06% chance (6 out of 10000) of being down between 0 and 88 BB rather than a 20% chance of being down that much or worse.

For the hell of it, let's take all these players and let them all play out the rest of their 15000 hands under non-bonus conditions. Obviously the players who have played the entire time without a bonus will be identical to the population from my last post - about 6.4% of them would still be down money. What about the group that played the bonus at the start? The details are boring and I'll omit them, but it turns out only 23 of those 10000 players will be down money at the end of 15000 hands. Again, I know which group I'd rather be in - the group where I have a 0.23% chance of losing a little bit of money rather than a 6.4% chance of losing that or more.

These numbers will obviously vary depending on what bonus multiplier and level you clear them at, but the message is undeniable. Playing a bonus doesn't actaully reduce your variance in real-dollar terms, but does raise your expectation enough that even a quite hefty variance can be borne. And I haven't even focused on the other (cheery) end of the distribution tail, whereby you make far more money under bonus that you ever could have without it!

My natural "instinct" before writing this post was to be a bonus chaser, and after writing this post it only reinforces that tendency. Given these numbers, I think I'm a LONG way off from playing at a level where I can afford to ignore the positive effects that I've outlined here.